Math Is Fun Forum
You are not logged in.
- Topics: Active | Unanswered
#1 Jokes » Love Jokes - IV » Today 13:50:40
- Jai Ganesh
- Replies: 0
Knock Knock.
Who's there?
Olive!
Olive who?
Olive you!
* * *
Knock Knock.
Who's there?
Owl.
Owl(s) who?
"Owl always love you".
* * *
The gods gave man fire and he invented fire engines.
They gave him love and he invented marriage.
* * *
True love is when your pet comes to your room on its own.
* * *
Roses are red, violets are blue, if you love Star Wars, may the force be with you.
* * *
#2 Re: Exercises » Compute the solution: » Today 13:34:15
Hi,
Good!
2576.
#3 Re: Dark Discussions at Cafe Infinity » crème de la crème » Yesterday 21:58:17
2337) Victor Ambrose
Gist:
Work
Our genome can be likened to an instruction manual for all cells in our body. Every cell contains the same set of instructions. Yet, different cell types have very distinct characteristics. These differences arise from gene regulation, which allows each cell to select only the relevant instructions. In 1993, Victor Ambros and Gary Ruvkun discovered microRNA, a new class of tiny RNA molecules that play a crucial role in gene regulation. This new dimension to gene regulation is fundamentally important for how organisms develop and function.
Summary
Victor Ambros (born December 1, 1953, Hanover, New Hampshire, U.S.) is an American developmental biologist and molecular geneticist best known for his pioneering work in the discovery of microRNA (miRNA), a type of small RNA molecule that serves essential functions in regulating gene expression. Ambros’s contributions to the discovery of miRNA had a profound impact on scientific understanding of cell function and mechanisms underlying gene activity and disease and was particularly important for the fields of molecular biology and developmental biology. For his discoveries, he was awarded the 2024 Nobel Prize for Physiology or Medicine (shared with American molecular biologist and geneticist Gary Ruvkun).
Education and early research
Ambros spent his youth in Vermont, where his parents encouraged his interest in science. After graduating from high school, he attended the Massachusetts Institute of Technology (MIT), where he earned a bachelor’s degree in biology in 1975. He remained at MIT to pursue a Ph.D. in genetics, working under the guidance of American virologist and Nobelist David Baltimore while carrying out research aimed at better understanding the genomic structure and replication of poliovirus. In 1979 Ambros completed a Ph.D. and continued on at MIT as a postdoctoral researcher in the laboratory of biologist H. Robert Horvitz. There he later worked with Ruvkun, who was also a postdoctoral student with Horvitz, to investigate genetic factors dictating the timing of events in the development of the nematode Caenorhabditis elegans.
Lin-4 and miRNA
In the late 1980s, after joining the faculty at Harvard University, Ambros studied more deeply a strain of C. elegans that carries a mutation in a gene known as lin-4. Lin-4 exerts temporal control over developmental events in C. elegans larvae by negatively regulating LIN-14 protein. In the course of their investigations, Ambros and his team realized that lin-4 produces only a very short strand of RNA, which is not translated into protein, and that lin-4 interacts with a gene known as lin-14. Although it was apparent to Ambros and others that lin-4 somehow regulates lin-14 activity, the mechanism was a mystery.
In 1992, while still trying to elucidate lin-4 regulatory mechanisms, Ambros moved his laboratory to Dartmouth College. The following year his laboratory published its findings on the short RNA produced by lin-4. He and Ruvkun then compared their insights on lin-4 and lin-14, which Ruvkun’s laboratory had been investigating, and found that the short lin-4 RNA sequence was complementary to a segment of lin-14 messenger RNA (mRNA). They also showed that binding of lin-4 RNA to lin-14 mRNA blocks LIN-14 protein production. Following the publication of their work, it was recognized that they had discovered not only a novel RNA molecule—miRNA—but also a previously unknown mechanism of gene regulation. In 2008 Ambros joined the faculty at the University of Massachusetts Medical School, where his research continues to center on characterizing the role of miRNA in development.
Awards and honors
Ambros has received numerous awards and honors throughout his career, including the 2008 Lasker Award (shared with Ruvkun and David C. Baulcombe), the 2008 Gairdner International Award (shared with Ruvkun), and the 2014 Wolf Prize (shared with Ruvkun and Nahum Sonenberg). Ambros is a fellow of the American Academy of Arts and Sciences (2011) and the American Association for the Advancement of Science (2018).
Details
Victor R. Ambros (born December 1, 1953) is an American developmental biologist who discovered the first known microRNA (miRNA). He is a professor at the University of Massachusetts Medical School. He completed both his undergraduate and doctoral studies at the Massachusetts Institute of Technology. Ambros received the Nobel Prize in Physiology or Medicine in 2024 for his research on microRNA.
Biography:
Early life and education
Ambros was born in New Hampshire. His father, Longin Ambros, attended Sigismund Augustus Gymnasium in Vilnius 1937-1939 and was a Polish World War II refugee. Victor grew up on a small dairy farm in Hartland, Vermont, in a family of eight children and attended Woodstock Union High School.
From the Massachusetts Institute of Technology, Ambros received a Bachelor of Science with a major in biology in 1975 and a Doctor of Philosophy in biology in 1979. His doctoral supervisor was David Baltimore, a 1975 Nobel laureate in Physiology or Medicine. Ambros continued his research at MIT as the first postdoctoral fellow in the lab of future Nobel laureate H. Robert Horvitz.
Career
Ambros became a faculty member at Harvard University in 1984. However, Harvard denied tenure to Ambros shortly after he discovered what is now known as microRNA. About this, Baltimore later said in 2008: "They lost a potential Nobel laureate because they simply didn’t see in him the potential that he had ... It’s the nature of a seminal discovery that it’s seminal in retrospect. You can’t know ahead of time."
Ambros joined the faculty of Dartmouth College in 1992. He joined the faculty at the University of Massachusetts Medical School in 2008, and currently holds the title of Silverman Professor of Natural Sciences in the program in Molecular Medicine, endowed by his former Dartmouth student, Howard Scott Silverman.
Research
In 1993, Ambros and his co-workers Rosalind Lee and Rhonda Feinbaum reported in the journal Cell that they had discovered single-stranded non-protein-coding regulatory RNA molecules in the organism C. elegans. Previous research, including work by Ambros and Horvitz, had revealed that a gene known as lin-4 was important for normal larval development of C. elegans, a nematode often studied as a model organism. Specifically, lin-4 was responsible for the progressive repression of the protein LIN-14 during larval development of the worm; mutant worms deficient in lin-4 function had persistently high levels of LIN-14 and displayed developmental timing defects.
Ambros and colleagues found that lin-4, unexpectedly, did not encode a regulatory protein. Instead, it gave rise to some small RNA molecules, 22 and 61 nucleotides in length, which Ambros called lin-4S (short) and lin-4L (long). Sequence analysis showed that lin-4S was part of lin-4L: lin-4L was predicted to form a stem-loop structure, with lin-4S contained in one of the arms, the 5' arm. Furthermore, Ambros, together with Gary Ruvkun (Harvard), discovered that lin-4S was partially complementary to several sequences in the 3' untranslated region of the messenger RNA encoding the LIN-14 protein. Ambros and colleagues hypothesized and later determined that lin-4 could regulate LIN-14 through binding of lin-4S to these sequences in the lin-14 transcript in a type of antisense RNA mechanism.
In 2000, another C. elegans small RNA regulatory molecule, let-7, was characterized by the Ruvkun lab and found to be conserved in many species, including vertebrates. These discoveries, among others, confirmed that Ambros had in fact discovered a class of small RNAs with conserved functions, now known as microRNA.
Ambros was elected to the United States National Academy of Sciences in 2007. He was elected a Fellow of the American Academy of Arts and Sciences in 2011. In 2024 he shared the Nobel Prize in Physiology and Medicine with Gary Ruvkun "for the discovery of microRNA and its role in post-transcriptional gene regulation".

#4 This is Cool » Magnet » Yesterday 17:06:38
- Jai Ganesh
- Replies: 0
Magnet
Gist
A magnet is a material or object that produces a magnetic field. This magnetic field is invisible but is responsible for the most notable property of a magnet: a force that pulls on other ferromagnetic materials, such as iron, steel, nickel, cobalt, etc. and attracts or repels other magnets.
A magnet is any object that produces its own magnetic field that interacts with other magnetic fields. Magnets have two poles, a north pole and a south pole. The magnetic field is represented by field lines that start at a magnet's north pole and end at the south pole.
Summary
A magnet is a material or object that produces a magnetic field. This magnetic field is invisible but is responsible for the most notable property of a magnet: a force that pulls on other ferromagnetic materials, such as iron, steel, nickel, cobalt, etc. and attracts or repels other magnets.
A permanent magnet is an object made from a material that is magnetized and creates its own persistent magnetic field. An everyday example is a refrigerator magnet used to hold notes on a refrigerator door. Materials that can be magnetized, which are also the ones that are strongly attracted to a magnet, are called ferromagnetic (or ferrimagnetic). These include the elements iron, nickel and cobalt and their alloys, some alloys of rare-earth metals, and some naturally occurring minerals such as lodestone. Although ferromagnetic (and ferrimagnetic) materials are the only ones attracted to a magnet strongly enough to be commonly considered magnetic, all other substances respond weakly to a magnetic field, by one of several other types of magnetism.
Ferromagnetic materials can be divided into magnetically "soft" materials like annealed iron, which can be magnetized but do not tend to stay magnetized, and magnetically "hard" materials, which do. Permanent magnets are made from "hard" ferromagnetic materials such as alnico and ferrite that are subjected to special processing in a strong magnetic field during manufacture to align their internal microcrystalline structure, making them very hard to demagnetize. To demagnetize a saturated magnet, a certain magnetic field must be applied, and this threshold depends on coercivity of the respective material. "Hard" materials have high coercivity, whereas "soft" materials have low coercivity. The overall strength of a magnet is measured by its magnetic moment or, alternatively, the total magnetic flux it produces. The local strength of magnetism in a material is measured by its magnetization.
An electromagnet is made from a coil of wire that acts as a magnet when an electric current passes through it but stops being a magnet when the current stops. Often, the coil is wrapped around a core of "soft" ferromagnetic material such as mild steel, which greatly enhances the magnetic field produced by the coil.
Details
A magnet is a special kind of metal which is made out of (Iron Nickel and Cobalt) When a magnet goes near a special kind of metal or another magnet, and the poles (sides) touching are opposite, it will pull, or attract the other object closer. If the two poles are the same, the magnet and the other object will push away, or repel, from each other. This attraction and repulsion is called magnetism. All magnets have north and south poles. Opposite poles are attracted to each other, while the same poles repel each other like south and south and north and north. When you rub a piece of iron along a magnet, the north-seeking poles of the atoms in the iron line up in the same direction. The force generated by the aligned atoms creates a magnetic field.
Types of magnet
Soft magnets (meaning impermanent magnets) are often used in electromagnets some of the magnets are (bar magnet,wand and ball magnet). These increase (often hundreds or thousands of times) the magnetic field of a wire that carries an electrical current and is wrapped around the magnet. The field also increases with the current. Magnetic Materials: Soft Magnets. Soft magnetic materials are those materials that are easily magnetised and demagnetised.
Permanent magnets have ferromagnetism. They occur naturally in some rocks, particularly lodestone, but are now commonly manufactured. A magnet's magnetism decreases when it is heated and increases when it is cooled. It has to be heated at around 1,000 degrees Celsius (1,830 °F). Like poles (S-pole and S-pole/N-pole and N-pole) will repel each other while unlike poles (N-pole and S-pole) will attract each other.
Magnets are only attracted to special metals. Iron, cobalt and nickel are magnetic. Metals that have iron in them attract magnets well. Steel is one. Metals like brass, copper, zinc and aluminium, silver are not attracted to magnets. Non-magnetic materials such as wood and glass are not attracted to magnets as they do not have magnetic materials in them.
Rare earth magnets
Lanthanum elements can make strong magnets. The spin of their electrons can be aligned, resulting in very strong magnetic fields. So these elements are used for high-strength magnets when their high price is not a concern. The most common types of rare-earth magnets are samarium–cobalt and neodymium–iron–boron (NIB) magnets.
Natural magnets
Natural/permanent magnets are not artificial. They are a kind of rock called lodestone or magnetite.
The compass
A compass uses the Earth's magnetic field, and points to the North magnetic pole. A north side of the magnet is attracted to the south side of another magnet. However, the north side of the compass points to the north pole, this can only mean that the "north pole" is really the magnetic south, and the "South magnetic pole" is really the magnetic north.
Discovery
Ancient people discovered magnetism from lodestones (or magnetite) which are naturally magnetized pieces of iron ore. Lodestones, suspended so they could turn, were the first magnetic compasses.
The earliest known surviving descriptions of magnets and their properties are from Anatolia, India, and China about 2500 years ago. The properties of lodestones and their affinity for iron were written of by Pliny the Elder in his encyclopedia Naturalis Historia.
Additional Information
A magnet is any material capable of attracting iron and producing a magnetic field outside itself. By the end of the 19th century all the known elements and many compounds had been tested for magnetism, and all were found to have some magnetic property. The most common was the property of diamagnetism, the name given to materials exhibiting a weak repulsion by both poles of a magnet. Some materials, such as chromium, showed paramagnetism, being capable of weak induced magnetization when brought near a magnet. This magnetization disappears when the magnet is removed. Only three elements, iron, nickel, and cobalt, showed the property of ferromagnetism (i.e., the capability of remaining permanently magnetized).
You probably know that magnets attract specific metals and they have north and south poles. Opposite poles attract each other while like poles repel each other. Magnetic and electrical fields are related, and magnetism, along with gravity and strong and weak atomic forces, is one of the four fundamental forces in the universe.
But none of those facts answers the most basic question: What exactly makes a magnet stick to certain metals? Or why don't they stick to other metals? Why do they attract or repel each other, depending on their positioning? And what makes neodymium magnets so much stronger than the ceramic magnets we played with as children?
To understand the answers to these questions, it helps to have a basic definition of a magnet. Magnets are objects that produce magnetic fields and attract metals like iron, nickel and cobalt. The magnetic field's lines of force exit the magnet from its north pole and enter its south pole. Permanent or hard magnets create their own magnetic field all the time. Temporary or soft magnets produce magnetic fields while in the presence of a magnetic field and for a short while after exiting the field. Electromagnets produce magnetic fields only when electricity travels through their wire coils.
Because electrons and protons are tiny magnets, all materials have some sort of magnetic property. In most materials, however, the way electrons spin in opposite directions cancels out an atom's magnetic properties. Metals are the most common choices to manufacture magnets. Although some are made from simple metals, combinations of metals — called alloys — produce magnets of different strengths. For example:
Ferrites or ceramic magnets: These are like those used in refrigerator magnets and elementary-school science experiments. They contain iron oxide and other metals in a ceramic composite. A ceramic magnet known as lodestone, or magnetite, was the first magnetic material discovered and occurs naturally. Even though the ceramic magnet has been around for so long, they weren't commercially produced until 1952. Although they're common and keep their magnetism, they tend to have a weaker magnetic field (known as the energy product) than other types of magnets.
Alnico magnets: These were developed in the 1930s and are made from aluminum, nickel and cobalt. They're stronger than ceramic magnets, but not as strong as the ones that incorporate a class of elements known as rare-earth metals.
Neodymium magnets: These contain iron, boron and the rare-earth element neodymium, and as of this writing, they are the strongest commercially available magnets. They first appeared in the 1980s after scientists at the General Motors Research Laboratories and the Sumitomo Special Metals Company published their research.
Samarium cobalt magnets: These were developed by scientists at the Dayton University Research University in the 1960s, and combine cobalt with the rare-earth element samarium. In the past few years, scientists have also discovered magnetic polymers, or plastic magnets. Some of these are flexible and moldable. However, some work only at extremely low temperatures, and others pick up only very lightweight materials, like iron filings.
The Basics
Many of today's electronic devices require magnets to function. This reliance on magnets is relatively recent, primarily because most modern devices require magnets that are stronger than the ones found in nature. Lodestone, a form of magnetite, is the strongest naturally occurring magnet. It can attract small objects, like paper clips and staples.
By the 12th century, people had discovered that they could use lodestone to magnetize pieces of iron, creating a compass. Repeatedly rubbing lodestone along an iron needle in one direction magnetized the needle. It would then align itself in a north-south direction when suspended. Eventually, scientist William Gilbert explained that this north-south alignment of magnetized needles was due to Earth behaving like an enormous magnet with north and south poles.
A compass needle isn't nearly as strong as many of the permanent magnets used today. But the physical process that magnetizes compass needles and chunks of neodymium alloy is essentially the same. It relies on microscopic regions known as magnetic domains, which are part of the physical structure of ferromagnetic materials, like iron, cobalt and nickel. Each domain is essentially a tiny, self-contained magnet with a north and south pole. In an unmagnetized ferromagnetic material, each domain's north pole points in a random direction. Magnetic domains that are oriented in opposite directions cancel one another out, so the material does not produce a net magnetic field.
In magnets, on the other hand, most or all the magnetic domains point in the same direction. Rather than canceling one another out, the microscopic magnetic fields combine to create one large magnetic field. The more domains point in the same direction, the stronger the overall field. Each domain's magnetic field extends from its north pole into the south pole of the domain ahead of it.
This explains why breaking a magnet in half creates two smaller magnets with north and south poles. It also explains why opposite poles attract — the field lines leave the north pole of one magnet and naturally enter the south pole of another, essentially creating one larger magnet. Like poles repel each other because their lines of force are traveling in opposite directions, clashing with each other rather than moving together.

#5 Re: This is Cool » Miscellany » Yesterday 16:38:30
2389) Vatican City
Gist
Vatican City is the world's smallest sovereign state, an independent city-state and the administrative center of the Roman Catholic Church, located as an enclave within Rome, Italy. Ruled by the Pope as the head of the Holy See, it is a site of immense religious and cultural importance, home to landmarks like St. Peter's Basilica, the Vatican Museums, and the Sistine Chapel. Established by the Lateran Treaty of 1929, it serves as the global spiritual headquarters for the Catholic Church and is a pilgrimage site for Christians worldwide.
Vatican City is famous for being the sovereign spiritual and administrative center of the Roman Catholic Church, the world's smallest independent state, and a major pilgrimage site. It is home to significant religious and cultural landmarks like St. Peter's Basilica and the Sistine Chapel with Michelangelo's frescoes, as well as the extensive Vatican Museums with its vast collection of art, all of which draw millions of tourists and pilgrims annually.
Summary
Vatican City, officially the Vatican City State, often shortened as the Vatican, is a landlocked sovereign state and city-state. Ruled by the pope, it is an enclave within Rome and serves as the administrative centre of the Catholic Church. Vatican City is governed by the See of Rome, commonly known as the Holy See, itself a sovereign entity under international law, which maintains its temporal power, governance, diplomacy, and spiritual independence. Vatican is also used as a metonym for the Holy See, which is the central governing body of the Catholic Church and Vatican City, comprising the pope and the Roman Curia. The independent state of Vatican City came into existence in 1929 via the Lateran Treaty between the Holy See and the Kingdom of Italy, which spoke of it as a new creation, not as a vestige of the much larger Papal States (756–1870), which had previously encompassed much of Central Italy.
With an area of 49 hectares (121 acres)[g] and a population of about 882 in 2024, it is the smallest sovereign state in the world both by area and by population. It is among the least populated capitals in the world. As governed by the Holy See, Vatican City State is an ecclesiastical or sacerdotal-monarchical state ruled by the pope, who is the bishop of Rome and head of the Catholic Church; the highest state functionaries are all Catholic clergy of various origins. The Holy See dates to early Christianity and is the principal episcopal see of the Catholic Church, which in 2018 had about 1.329 billion baptized Catholics in the world, in the Latin Church and 23 Eastern Catholic Churches. After the Avignon Papacy (1309–1377) the popes have mainly resided at the Apostolic Palace within what is now Vatican City, although at times residing instead in the Quirinal Palace in Rome or elsewhere.
Vatican City contains religious and cultural sites such as St Peter's Basilica, the Sistine Chapel, the Vatican Apostolic Library, and the Vatican Museums. They feature some of the world's most famous paintings and sculptures. The economy of Vatican City is supported financially by donations from Catholic believers, by the sale of postage stamps and souvenirs, fees for admission to museums, and sales of publications. Vatican City has no taxes, and items are duty-free.
Details
Vatican City is a landlocked ecclesiastical state, seat of the Roman Catholic Church, and an enclave surrounded by Rome, situated on the west bank of the Tiber River. Vatican City is the world’s smallest fully independent nation-state.
Layout of the city
Vatican City’s medieval and Renaissance walls form its boundaries, except on the southeast at St. Peter’s Square (Piazza San Pietro). Of the six entrances, only three—the piazza, the Arco delle Campane (Arch of the Bells) in the facade of St. Peter’s Basilica, and the entrance to the Vatican Museums and Galleries in the north wall—are open to the public. The most imposing building is St. Peter’s Basilica, built during the 4th century and rebuilt during the 16th century. Erected over the tomb of St. Peter the Apostle, it is the second largest religious building (after Yamoussoukro Basilica) in Christendom.
The Vatican Palace is the residence of the pope within the city walls. The Holy See is the name given to the government of the Roman Catholic Church, which is led by the pope as the bishop of Rome. As such, the Holy See’s authority extends over Catholics throughout the world. Since 1929 it has resided in Vatican City, which was established as an independent state to enable the pope to exercise his universal authority.
Vatican City has its own telephone system, post office, gardens, astronomical observatory, radio station, banking system, and pharmacy, as well as a contingent of Swiss Guards responsible for the personal safety of the pope since 1506. Almost all supplies—including food, water, electricity, and gas—must be imported. There is no income tax and no restriction on the import or export of funds. As the Holy See, it derives its income from the voluntary contributions of more than one billion Roman Catholics worldwide, as well as interest on investments and the sale of stamps, coins, and publications. Banking operations and expenditures have been reported publicly since the early 1980s.
History and governance
The city of Rome has been an important center of Christianity since the early days of the church. St. Peter, considered the first pope, is thought to have lived and died in Rome. In 313 Emperor Constantine I issued the Edict of Milan, which ended official persecution of Christians and opened the door to the growth of the church in both spiritual and material terms. By the 4th century the church had gained control of a great deal of territory, called the Patrimony of St. Peter, in and around Rome. Papal influence in central Italy began to increase in the 5th century, as the Roman Empire fell apart and the people of the area began to rely on the pope for protection from invading armies. By about the year 600 the church was one of the largest landowners in the world.
The legal basis for the foundation of the Papal States was provided by the Donation of Pippin, which granted the pope the rights to large parts of central Italy in 754. In the 9th century the first city walls (Leonine Walls) were completed under Pope Leo IV. Between the 12th and 14th centuries the Vatican underwent something of a building boom as a new palace was built and the Leonine Walls were restored. The Vatican fell into decay after 1309, when the office of the papacy was moved to Avignon in France. The pope’s return to Rome in 1377 marked the beginning of a revitalization.
Italy became a unified country in the 19th century, which led to major changes in the Vatican’s political status. Most immediately, the church lost its land to the new country. Some of the papal territories voted to join the Kingdom of Sardinia in 1859. Italy annexed the rest of the Papal States by 1870 and made Rome the Italian capital. To protest the incorporation into a unified Italy, each pope thereafter remained a voluntary “prisoner of the Vatican,” never leaving the small territory of the papal grounds. This situation lasted nearly 60 years.
In 1929 a solution to this ongoing problem was found. Vatican City’s independent sovereignty was recognized by the Fascist Italian government in the Lateran Treaty. Sovereignty is exercised by the pope upon his election as the head of the Roman Catholic Church. He has absolute executive, legislative, and judicial powers within the city. While most of the inhabitants of Vatican City are priests or nuns, they also include several hundred laypersons engaged in secretarial, domestic, trade, and service occupations.
Institutions and attractions
Special extraterritorial privileges are extended to more than 10 other buildings in Rome and to Castel Gandolfo, the pope’s summer residence in the Alban Hills. In addition, Vatican City maintains embassies in numerous foreign nations.
The Vatican enjoyed a cultural golden age during the Renaissance, when the popes were among Italy’s foremost patrons of the arts. The Vatican Museums and Galleries, the frescoes by Michelangelo in the Sistine Chapel, the frescoes by Pinturicchio in the Borgia Apartment, and Raphael’s Stanze (“Rooms”) attract critics, artists, and flocks of tourists from throughout the world. Years of restoration work on the Sistine Chapel frescoes were completed in 1994, making it possible to view Michelangelo’s work in full vibrant colors. In 2000 the millennial Jubilee focused world attention on Vatican City.
The Vatican Apostolic Library contains a priceless collection of some 150,000 manuscripts and 1.6 million printed books, many from pre-Christian and early Christian times. The Vatican publishes its own influential daily newspaper, L’Osservatore Romano, and its press can print books and pamphlets in any of 30 languages, from old Ecclesiastical Georgian to Tamil. Since 1983 the Vatican has produced its own television programming. Its radio broadcasts are heard in some 40 languages in many parts of the world. Vatican City was designated a UNESCO World Heritage site in 1984. Pop. (2019 est.) 453.
Additional Information
The Vatican City, one of the most sacred places in Christendom, attests to a great history and a formidable spiritual venture. A unique collection of artistic and architectural masterpieces lie within the boundaries of this small state. At its centre is St Peter's Basilica, with its double colonnade and a circular piazza in front and bordered by palaces and gardens. The basilica, erected over the tomb of St Peter the Apostle, is the largest religious building in the world, the fruit of the combined genius of Bramante, Raphael, Michelangelo, Bernini and Maderno.
Outstanding Universal Value:
Brief synthesis
One of the most sacred places in Christendom, Vatican City stands as a testimony to a history of about two millennia and to a formidable spiritual venture. Site of the tomb of the Apostle Saint Peter, first of the uninterrupted succession of Roman Pontiffs, and therefore a main pilgrimage centre, the Vatican is directly and tangibly linked with the history of Christianity. Furthermore, it is both an ideal and an exemplary creation of the Renaissance and of Baroque art. It exerted an enduring influence on the development of the arts from the 16th century.
The independent State, defined by the Lateran Treaty of 11 February 1929, extends its territorial sovereignty over an area of 44 ha in the centre of Rome: Vatican City enclosed by its walls and open toward the city through Bernini’s colonnade of Saint Peter’s. The boundaries of the city-state contain masterpieces and living institutions that are a witness to the unique continuity of the crucial role played by this place in the history of mankind. The Centre of Christianity since the foundation of Saint Peter’s Basilica by Constantine (4th century), and at a later stage the permanent seat of the Popes, the Vatican is at once the pre-eminently holy city for Catholics, an important archaeological site of the Roman world and one of the major cultural reference points of both Christians and non-Christians.
Its prestigious history explains the development of an architectural and artistic ensemble of exceptional value. Beneath the basilica of Saint Peter, reconstructed in the 16th century under the guidance of the most brilliant architects of the Renaissance, remains of the first basilica founded by Constantine still exist, as well as ruins of the circus of Caligula and Nero, and a Roman necropolis of the 1st century AD, where Saint Peter’s tomb is located. Under Julius II’s patronage in 1506, an extraordinary artistic era was inaugurated, leading to the decoration of Raphael’s Stanze and of the Sistine Chapel with frescoes by Michelangelo, along with the building of the new basilica, completed in 1626, fruit of the combined genius of Bramante, Raphael, Michelangelo, Bernini, Maderno and Della Porta.
The Vatican Palace is the result of a long series of additions and modifications by which, from the Middle Ages, the Popes rivalled each other in magnificence. The original building of Nicholas III (1277-1280) was enlarged in the 15th, 16th and 17th centuries: the history of the arts of the Renaissance and Baroque periods finds here iconic models.
In 1475, Sixtus IV founded the Vatican Library, which is the first open to the public in Europe; the collections of manuscripts and books, prints, drawings, coins and decorative arts, constantly increased through the centuries, making it an invaluable repository of human culture.
From the mid-18th century, the popes’ efforts were also directed towards expanding the private collections of antiquities dating back to the Renaissance: their transformation into public museums accessible to scholars and connoisseurs marks the origin of the Vatican Museums. New buildings were built specifically to house the classical sculptures, such as the Pio-Clementine Museum, which represents a milestone in the history of European culture. The 19th- and 20th-century additions of new and diverse collections and buildings accord with the tradition of papal patronage.
Criterion (i): The Vatican, a continuous artistic creation whose progress spreads over centuries, represents a unique masterpiece of the modelling of a space, integrating creations which are among the most renowned of mankind: not only the world famous icon of sacred architecture, the basilica of Saint Peter, but also the chapel of Nicholas V decorated by Fra Angelico, the Borgia apartment with frescoes by Pinturicchio, the Stanze of Raphael and his students, the Sistine Chapel, whose mural decoration, begun by Perugino, Botticelli and other painters, was completed in the 16th century with the frescoes of the ceiling and the monumental Last Judgement by Michelangelo, who left his last murals in the Pauline Chapel.
Criterion (ii): The Vatican exerted a deep influence on the development of art from the 16th century. Architects have visited it to study the constructions of Bramante (the Basilica of Saint Peter, the Belvedere Court), of Michelangelo (the cupola of Saint Peter), of Bernini (Saint Peter's colonnade, the Baldacchino of the Basilica). Both within and outside Europe, the Vatican buildings have been abundantly copied and imitated, the paintings (the frescoes of Raphael and Michelangelo) and the antiquities of the Museums no less so.
Criterion (iv): The Vatican is both an ideal and exemplary religious and palatial creation of the Renaissance and of Baroque art.
Criterion (vi): Site of the tomb of Saint Peter and pilgrimage centre, the Vatican is directly and materially linked with the history of Christianity. For more than a thousand years, mankind has accumulated, in this privileged site, the treasures of its collective memory (manuscripts and books of the Library) and of its universal genius.
Integrity
The boundaries of the property, which coincide with the entire territory of the Vatican City State, have preserved their original integrity and characteristics. The exceptional urban, architectural and aesthetic values, even through successive additions and changes in form and design, invariably maintain the highest standards of artistic quality and workmanship, building an organic ensemble of unparalleled harmony. Civil and sacred buildings, which have been in use for centuries, maintain their religious, cultural, institutional and diplomatic functions unaltered.
Authenticity
The property meets the required conditions of authenticity, since most of its features are still preserved and maintained in their initial form, perform their primary functions and truthfully convey their original spiritual and cultural values. The extensive restoration campaigns conducted on some of the most significant monuments of the site since the date of the inscription ensure the material conservation of the heritage and strengthen its capacity for expressing its values.
Protection and management requirements
The property is safeguarded by the law for the protection of the cultural heritage (no. 355, 25/07/2001) and by several rules of procedure issued by the various institutions of the Holy See in charge of heritage. For instance, the body responsible for the preservation and maintenance of Saint Peter’s Basilica, the Fabbrica di S.Pietro, was founded in 1506 and is still active. The legal protective mechanism and traditional management system are adequate and ensure the effective protection of the site. The state of conservation of the property is constantly and carefully monitored, with special attention paid to the impact of the huge number of pilgrims and visitors.

#6 Dark Discussions at Cafe Infinity » Close Quotes - VII » Yesterday 15:38:47
- Jai Ganesh
- Replies: 0
Close Quotes - VII
1. I can't do with mountains at close quarters - they are always in the way, and they are so stupid, never moving and never doing anything but obtrude themselves. - D. H. Lawrence
2. There's no doubt who was a leader in space after the Apollo Program. Nobody came close to us. And our education system, in science, technology, engineering and math, was at the top of the world. It's no longer there. We're descending rather rapidly. - Buzz Aldrin
3. One of the best ways to see tree flowers is to climb one of the tallest trees and to get into close, tingling touch with them, and then look broad. - John Muir
4. Close by the Rights of Man, at the least set beside them, are the Rights of the Spirit. - Victor Hugo
5. It's a brilliant surface in that sunlight. The horizon seems quite close to you because the curvature is so much more pronounced than here on earth. It's an interesting place to be. I recommend it. - Neil Armstrong
6. No evidence compels the conclusion that the minimum required intake of any vitamin comes close to the optimum intake that sustains good health. - Linus Pauling
7. Before I lost my voice, it was slurred, so only those close to me could understand, but with the computer voice, I found I could give popular lectures. I enjoy communicating science. It is important that the public understands basic science, if they are not to leave vital decisions to others. - Stephen Hawking
8. It's interesting that I had such a close relationship with my grandfather. Because your parents always judge you: they say, 'You shouldn't do this, you shouldn't do that.' But with your grandparents you have a feeling that you can say anything or you can do anything, and they will support you. That's why you have this kind of connection. - Novak Djokovic.
#7 Science HQ » Polonium » Yesterday 15:18:51
- Jai Ganesh
- Replies: 0
Polonium
Gist
Polonium (Po, atomic number 84) is a rare, highly radioactive metal discovered by Marie and Pierre Curie in 1898. It has no stable isotopes, with Polonium-210 (210Po) being the most common, formed as a decay product of uranium-238. Although Po-210 poses no external hazard, internal contamination from inhalation or ingestion can cause significant internal radiation doses, leading to severe health effects or death. Its industrial applications include the removal of static electricity in industries like paper and metal rolling.
Polonium has limited industrial uses, including antistatic devices, heat sources for space equipment, and neutron sources for research and nuclear applications. However, its extreme radioactivity and short half-life make it dangerous and challenging to handle, with potential applications for poisoning and in the development of atomic weapons.
Summary
Polonium is a chemical element; it has symbol Po and atomic number 84. A rare and highly radioactive metal (although sometimes classified as a metalloid) with no stable isotopes, polonium is a chalcogen and chemically similar to selenium and tellurium, though its metallic character resembles that of its horizontal neighbors in the periodic table: thallium, lead, and bismuth. Due to the short half-life of all its isotopes, its natural occurrence is limited to tiny traces of the fleeting polonium-210 (with a half-life of 138 days) in uranium ores, as it is the penultimate daughter of natural uranium-238. Though two longer-lived isotopes exist (polonium-209 with a half-life of 124 years and polonium-208 with a half-life of 2.898 years), they are much more difficult to produce. Today, polonium is usually produced in milligram quantities by the neutron irradiation of bismuth. Due to its intense radioactivity, which results in the radiolysis of chemical bonds and radioactive self-heating, its chemistry has mostly been investigated on the trace scale only.
Polonium was discovered on 18 July 1898 by Marie Skłodowska-Curie and Pierre Curie, when it was extracted from the uranium ore pitchblende and identified solely by its strong radioactivity: it was the first element to be discovered in this way. Polonium was named after Marie Skłodowska-Curie's homeland of Poland, which at the time was partitioned between three countries. Polonium has few applications, and those are related to its radioactivity: heaters in space probes, antistatic devices, sources of neutrons and alpha particles, and poison (e.g., poisoning of Alexander Litvinenko). It is extremely dangerous to humans.
Details
Polonium (Po) is a radioactive, silvery-gray or black metallic element of the oxygen group (Group 16 [VIa] in the periodic table). The first element to be discovered by radiochemical analysis, polonium was discovered in 1898 by Pierre and Marie Curie, who were investigating the radioactivity of a certain pitchblende, a uranium ore. The very intense radioactivity not attributable to uranium was ascribed to a new element, named by them after Marie Curie’s homeland, Poland. The discovery was announced in July 1898. Polonium is extremely rare, even in pitchblende: 1,000 tons of the ore must be processed to obtain 40 milligrams of polonium. Its abundance in the Earth’s crust is about one part in {10}^{15}. It occurs in nature as a radioactive decay product of uranium, thorium, and actinium. The half-lives of its isotopes range from a fraction of a second up to 103 years; the most common natural isotope of polonium, polonium-210, has a half-life of 138.4 days.
Polonium usually is isolated from by-products of the extraction of radium from uranium minerals. In the chemical isolation, pitchblende ore is treated with hydrochloric acid, and the resulting solution is heated with hydrogen sulfide to precipitate polonium monosulfide, PoS, along with other metal sulfides, such as that of bismuth, Bi2S3, which resembles polonium monosulfide closely in chemical behaviour, though it is less soluble. Because of the difference in solubility, repeated partial precipitation of the mixture of sulfides concentrates the polonium in the more soluble fraction, while the bismuth accumulates in the less soluble portions. The difference in solubility is small, however, and the process must be repeated many times to achieve a complete separation. Purification is accomplished by electrolytic deposition. It can be produced artificially by bombarding bismuth or lead with neutrons or with accelerated charged particles.
Chemically, polonium resembles the elements tellurium and bismuth. Two modifications of polonium are known, an α- and a β-form, both of which are stable at room temperature and possess metallic characteristics. The fact that its electrical conductivity decreases as the temperature increases places polonium among the metals rather than the metalloids or nonmetals.
Because polonium is highly radioactive—it disintegrates to a stable isotope of lead by emitting alpha rays, which are streams of positively charged particles—it must be handled with extreme care. When contained in such substances as gold foil, which prevent the alpha radiation from escaping, polonium is used industrially to eliminate static electricity generated by such processes as paper rolling, the manufacture of sheet plastics, and the spinning of synthetic fibres. It is also used on brushes for removing dust from photographic film and in nuclear physics as a source of alpha radiation. Mixtures of polonium with beryllium or other light elements are used as sources of neutrons.
Element Properties
atomic number : 84
atomic weight : 210
melting point : 254 °C (489 °F)
boiling point : 962 °C (1,764 °F)
density : 9.4 g/{cm}^{3}
oxidation states : −2, +2, +3(?), +4, +6.
Additional Information:
Appearance
A silvery-grey, radioactive semi-metal.
Uses
Polonium is an alpha-emitter, and is used as an alpha-particle source in the form of a thin film on a stainless steel disc. These are used in antistatic devices and for research purposes.
A single gram of polonium will reach a temperature of 500°C as a result of the alpha radiation emitted. This makes it useful as a source of heat for space equipment.
It can be mixed or alloyed with beryllium to provide a source of neutrons.
Biological role
Polonium has no known biological role. It is highly toxic due to its radioactivity.
Natural abundance
Polonium is a very rare natural element. It is found in uranium ores but it is uneconomical to extract it. It is obtained by bombarding bismuth-209 with neutrons to give bismuth-210, which then decays to form polonium. All the commercially produced polonium in the world is made in Russia.
#8 Re: Jai Ganesh's Puzzles » General Quiz » Yesterday 14:53:48
Hi,
#10557. What does the term in Geography Bourne (stream) mean?
#10558. What does the term in Geography Box canyon mean?
#9 Re: Jai Ganesh's Puzzles » English language puzzles » Yesterday 14:41:03
Hi,
#5751. What does the verb (used with object) despise mean?
#5752. What does the noun despite mean?
#10 Re: Jai Ganesh's Puzzles » Doc, Doc! » Yesterday 14:13:37
Hi,
#2469. What does the medical term Macrophage signify?
#11 Re: Jai Ganesh's Puzzles » 10 second questions » Yesterday 14:04:30
Hi,
#9735.
#12 Re: Jai Ganesh's Puzzles » Oral puzzles » Yesterday 13:52:31
Hi,
#6242.
#13 Jokes » Love Jokes - III » Yesterday 13:44:12
- Jai Ganesh
- Replies: 0
Q: Did you hear about the love affair between sugar and cream?
A: It was icing on the cake.
* * *
Q: How do you transfer funds even faster than electronic banking?
By getting Married!
* * *
Q: What happened when two vampires went on a blind date?
A: It was love at first bite!
* * *
Q: What's the difference between love and herpes?
A: Love doesn't last forever.
* * *
After a quarrel, a husband said to his wife, "You know, I was a fool when I married you."
She replied, "Yes, dear, but I was in love and didn't notice."
* * *
#14 Re: Exercises » Compute the solution: » Yesterday 13:28:11
Hi,
2575.
#15 Re: Dark Discussions at Cafe Infinity » crème de la crème » 2025-09-15 21:50:24
2336) John M. Jumper
Gist:
Work
Proteins control and drive all the chemical reactions that together are the basis of life. Proteins generally consist of 20 different amino acids. These are linked together in long strings that fold up to make a three-dimensional structure. In 2020, John Jumper and Demis Hassabis presented an AI model called AlphaFold2. With its help, they have been able to predict the structure of virtually all known proteins. AlphaFold2 has been widely used in many areas, including research into pharmaceuticals and environmental technology.
Summary
John M. Jumper (born 1985, Little Rock, Arkansas, U.S.) is an American computer scientist who was awarded the 2024 Nobel Prize in Chemistry for his work using artificial intelligence (AI) to find the three-dimensional structure of proteins. He shared half the prize with his colleague, English computer scientist Demis Hassabis, and the other half of the prize was awarded to American biochemist David Baker.
Early life and career
Jumper spent his childhood and teenage years in Little Rock, Arkansas. He majored in mathematics and physics at Vanderbilt University and graduated with a bachelor’s degree in 2007. He went on to complete a master’s degree in physics at the University of Cambridge in 2008.
Jumper spent the next three years at D.E. Shaw Research, a computational laboratory in New York City, where he developed simulations to examine the dynamics of proteins and other molecules. Starting in 2011 Jumper shifted his study of computational biology to the University of Chicago, where he applied machine learning to explore the physics of protein folding. He graduated from the University of Chicago with a master’s degree (2012) and a Ph.D. (2017) in chemistry. He then joined Google’s DeepMind, an AI development company, in 2017 as a research scientist working on AlphaFold, which predicts the three-dimensional structure of proteins using machine-learning algorithms.
Solving protein folding
Proteins are large molecules that are directly involved in the chemical processes essential for life and are built up from 20 amino acids that can be combined in many different ways. The function of a protein is determined by its three-dimensional structure, which can be quite complex based on how the string of amino acids is folded.
How a protein is folded is determined by its amino acid sequence. However, even a small protein of only 100 amino acids can have 1047 possible three-dimensional structures. Predicting a protein’s structure from its amino acid sequence became a key problem in molecular biology.
In 1994 biologists John Moult and Krzysztof Fidelis founded the Critical Assessment of protein Structure Prediction (CASP) challenge to test methods for predicting protein structures. Every two years, contestants were given the amino acid sequences for proteins whose structure had been determined but not published and were challenged to predict the protein structures.
Progress was slow. By the mid-2010s the best models in the CASP challenge were about 40 percent accurate. DeepMind entered its protein structure program AlphaFold in CASP13 in 2018 and delivered an astonishing accuracy of about 60 percent, far ahead of any competitors. However, improvement beyond that was difficult, but by then Jumper had joined DeepMind and used his experience with protein simulation to help develop AlphaFold2.
AlphaFold2 was trained on databases of amino acid sequences and protein structures and used a neural network called a transformer to find a likely protein structure. At CASP14 in 2020 AlphaFold2 reached an accuracy of 90 percent, which is comparable with experimental results. The problem of finding a protein structure given an amino acid sequence had been solved.
Jumper, DeepMind CEO Hassabis, and their collaborators used AlphaFold2 to calculate the structure of almost all of the more than 50,000 human proteins in 2021. They then went even further and calculated the structures of almost all of the 200 million known proteins, which come from about 1 million different species, or as Hassabis called it, “the entire protein universe.” By predicting how proteins organize themselves, researchers can develop more effective drugs that target specific proteins whose structures contribute to diseases. Since 2023 Jumper has been the director of Google DeepMind.
Jumper is also the recipient of the Wiley Prize in Biomedical Sciences (2022) and the BBVA Foundation Frontiers of Knowledge Award in Biology and Biomedicine (2023, shared with Hassabis and Baker). Jumper also received the VinFuture Prize (2022), the Canada Gairdner International Award (2023), and the Albert Lasker Basic Medical Research Award (2023, shared with Hassabis).
Details
John Michael Jumper (born 1985) is an American chemist and computer scientist. Jumper and Demis Hassabis were awarded with the 2024 Nobel Prize in Chemistry for protein structure prediction.
He currently serves as director at Google DeepMind. Jumper and his colleagues created AlphaFold, an artificial intelligence (AI) model to predict protein structures from their amino acid sequence with high accuracy. Jumper stated that the AlphaFold team plans to release 100 million protein structures.
The scientific journal Nature included Jumper as one of the ten "people who mattered" in science in their annual listing of Nature's 10 in 2021.
Education
Jumper received a Bachelor of Science with majors in physics and mathematics from Vanderbilt University in 2007, a Master of Philosophy in theoretical condensed matter physics from the University of Cambridge in 2010 on a Marshall Scholarship, a Master of Science in theoretical chemistry from the University of Chicago in 2012, and a Doctor of Philosophy in theoretical chemistry from the University of Chicago in 2017. His doctoral advisors at the University of Chicago were Tobin R. Sosnick and Karl Freed.
Career
Jumper's research investigates algorithms for protein structure prediction.
AlphaFold
AlphaFold is a deep learning algorithm developed by Jumper and his team at DeepMind, a research lab acquired by Google's parent company Alphabet Inc. It is an artificial intelligence program which performs predictions of protein structure.
Awards and honors
In November 2020, AlphaFold was named the winner of the 14th Critical Assessment of Structure Prediction (CASP) competition. This international competition benchmarks algorithms to determine which one can best predict the 3D structure of proteins. AlphaFold won the competition, outperforming other algorithms scoring above 90 for around two-thirds of the proteins in CASP's global distance test (GDT), a test that measures the degree to which a computational program predicted structure is similar to the lab experiment determined structure, with 100 being a complete match, within the distance cutoff used for calculating GDT.
In 2021, Jumper was awarded the BBVA Foundation Frontiers of Knowledge Award in the category "Biology and Biomedicine". In 2022 Jumper received the Wiley Prize in Biomedical Sciences and for 2023 the Breakthrough Prize in Life Sciences for developing AlphaFold, which accurately predicts the structure of a protein. In 2023 he was awarded the Canada Gairdner International Award and the Albert Lasker Award for Basic Medical Research.
In 2024, Jumper and Demis Hassabis shared half of the Nobel Prize in Chemistry for their protein folding predictions, the other half went to David Baker for computational protein design.
In 2025, Jumper received the Golden Plate Award of the American Academy of Achievement and the Marshall Medal of the Marshall Aid Commemoration Commission. He was elected a Fellow of the Royal Society in 2025.

#16 Re: Dark Discussions at Cafe Infinity » Greatest Mathematicians from 1 CE ... » 2025-09-15 21:26:51
22) Niccolò Fontana Tartaglia
Nicolo, known as Tartaglia (1499/1500 – 13 December 1557), was an Italian mathematician, engineer (designing fortifications), a surveyor (of topography, seeking the best means of defense or offense) and a bookkeeper from the then Republic of Venice. He published many books, including the first Italian translations of Archimedes and Euclid, and an acclaimed compilation of mathematics. Tartaglia was the first to apply mathematics to the investigation of the paths of cannonballs, known as ballistics, in his Nova Scientia (A New Science, 1537); his work was later partially validated and partially superseded by Galileo's studies on falling bodies. He also published a treatise on retrieving sunken ships.
Personal life
Nicolo was born in Brescia, the son of Michele, a dispatch rider who travelled to neighbouring towns to deliver mail. In 1506, Michele was murdered by robbers, and Nicolo, his two siblings, and his mother were left impoverished. Nicolo experienced further tragedy in 1512 when King Louis XII's troops invaded Brescia during the War of the League of Cambrai against Venice. The militia of Brescia defended their city for seven days. When the French finally broke through, they took their revenge by massacring the inhabitants of Brescia. By the end of battle, over 45,000 residents were killed. During the massacre, Nicolo and his family sought sanctuary in the local cathedral. But the French entered and a soldier sliced Nicolo's jaw and palate with a saber and left him for dead. His mother nursed him back to health but the young boy was left with a speech impediment, prompting the nickname "Tartaglia" ("stammerer"). After this he would never shave, and grew a beard to camouflage his scars.
His surname at birth, if any, is disputed. Some sources have him as "Niccolò Fontana", but others claim that the only support for this is a will in which he named a brother, Zuampiero Fontana, as heir, and point out that this does not imply he had the same surname.
#17 This is Cool » Aniline » 2025-09-15 18:38:35
- Jai Ganesh
- Replies: 0
Aniline
Gist
Aniline, or benzenamine (C6H5NH2), is the simplest aromatic amine, an industrially important organic compound used to make dyes, rubber, plastics, and other chemicals. It is a colorless to brown, oily liquid that darkens on exposure to air and light and has a characteristic odor. Aniline is a weak base, easily absorbed by the skin, and is a combustible liquid that can form flammable vapor/air mixtures and produce toxic fumes when heated or burned.
Aniline was first obtained in 1826 by the destructive distillation of indigo. Its name is taken from the specific name of the indigo-yielding plant Indigofera anil (Indigofera suffruticosa); its chemical formula is C6H5NH2.
Aniline is a chemical intermediate used to make a wide range of products, including dyes, pharmaceuticals (like paracetamol and Tylenol), polyurethane plastics, and rubber products like tires. It is also used in the agricultural industry to produce pesticides and fungicides.
Summary
Aniline (From Portuguese: anil, meaning 'indigo shrub', and -ine indicating a derived substance) is an organic compound with the formula C6H5NH2. Consisting of a phenyl group (−C6H5) attached to an amino group (−NH2), aniline is the simplest aromatic amine. It is an industrially significant commodity chemical, as well as a versatile starting material for fine chemical synthesis. Its main use is in the manufacture of precursors to polyurethane, dyes, and other industrial chemicals. Like most volatile amines, it has the odor of rotten fish. It ignites readily, burning with a smoky flame characteristic of aromatic compounds. It is toxic to humans.
Relative to benzene, aniline is "electron-rich". It thus participates more rapidly in electrophilic aromatic substitution reactions. Likewise, it is also prone to oxidation: while freshly purified aniline is an almost colorless oil, exposure to air results in gradual darkening to yellow or red, due to the formation of strongly colored, oxidized impurities. Aniline can be diazotized to give a diazonium salt, which can then undergo various nucleophilic substitution reactions.
Like other amines, aniline is both a base (pKaH = 4.6) and a nucleophile, although less so than structurally similar aliphatic amines.
Because an early source of the benzene from which they are derived was coal tar, aniline dyes are also called coal tar dyes.
Details
Aniline is an organic base used to make dyes, drugs, explosives, plastics, and photographic and rubber chemicals.
Aniline was first obtained in 1826 by the destructive distillation of indigo. Its name is taken from the specific name of the indigo-yielding plant Indigofera anil (Indigofera suffruticosa); its chemical formula is C6H5NH2.
Aniline is prepared commercially by the catalytic hydrogenation of nitrobenzene or by the action of ammonia on chlorobenzene. The reduction of nitrobenzene can also be carried out with iron borings in aqueous acid.
A primary aromatic amine, aniline is a weak base and forms salts with mineral acids. In acidic solution, nitrous acid converts aniline into a diazonium salt that is an intermediate in the preparation of a great number of dyes and other organic compounds of commercial interest. When aniline is heated with organic acids, it gives amides, called anilides, such as acetanilide from aniline and acetic acid. Monomethylaniline and dimethylaniline can be prepared from aniline and methyl alcohol. Catalytic reduction of aniline yields cyclohexylamine. Various oxidizing agents convert aniline to quinone, azobenzene, nitrosobenzene, p-aminophenol, and the phenazine dye aniline black.
Pure aniline is a highly poisonous, oily, colourless substance with a pleasant odour.
Additional Information
Aniline is the simplest member of the primary aromatic amines, in which one or more hydrogen atoms of the benzene ring are replaced by amino (-NH2) group.
Derivatives of aniline include a wide variety of different substances. Some of these (like benzidine and MOCA) are composed of two combined aromatic rings.
Many aromatic amines may cause methemoglobinemia in humans. Aniline and many of its derivatives are known or suspected human carcinogens. Several aniline derivatives can also cause skin sensitization. Classical members of this family are bladder carcinogens 2-naphtylamine and benzidine, both of which have been restricted in the European Union (EU) implying that there is no exposure to these compounds.
A large number of substances in the aniline group are on the market in the EU. Several aniline derivatives can be found also from the list of substances restricted under REACH. Aniline compounds are also formed as degradation products from azo-colourants, pharmaceuticals and from aromatic isocyanates used for polyurethane polymers, lacquers, foams and adhesives.
When looking at those aniline substances that are produced or imported in the EU at amounts above 1,000 tonnes per year (tpa) according to the European Chemical Agency’s (ECHA) registration database and that have significant health hazards, (other than only irritation/corrosion).

#18 Re: This is Cool » Miscellany » 2025-09-15 18:11:50
2388) Gulf
Gulf
"Gulf" refers to a large area of sea that is partially enclosed by land, such as the Gulf of Mexico, or a wide, deep chasm or separation between things, like the "gulf between rich and poor". The term can also refer to the famous Gulf Oil company, known for its lubricants and lubricants for vehicles.
A gulf is a portion of the ocean that penetrates land. Gulfs vary greatly in size, shape, and depth. They are generally larger and more deeply indented than bays. Like bays, they often make excellent harbors.
Summary
A gulf is a large inlet from an ocean or their seas into a landmass, larger and typically (though not always) with a narrower opening than a bay. The term was used traditionally for large, highly indented navigable bodies of salt water that are enclosed by the coastline. Many gulfs are major shipping areas, such as the Persian Gulf, Gulf of Mexico, Gulf of Finland, and Gulf of Aden.
Geographical Meaning
A gulf is a coastal indentation or a portion of the ocean or sea that penetrates the land.
It is typically larger and more deeply indented than a bay.
Examples include the Persian Gulf, the Gulf of Aden, and the Gulf of Finland.
Figurative Meaning
The term "gulf" can describe a wide or impassable separation, or a significant difference between two things or groups.
For instance, there is a widening gulf between different social classes.
Details
A gulf is a portion of the ocean that penetrates land. Gulfs vary greatly in size, shape, and depth. They are generally larger and more deeply indented than bays. Like bays, they often make excellent harbors. Many important trading centers are located on gulfs.
Gulfs may be formed by movements in Earth's crust. The planet's tectonic plates may rift, or break apart, creating a gulf. Or one plate may fold under another, a process called subduction. Subduction may create a gulf by making downfolds, or troughs, in the rock under the ocean.
Gulfs are sometimes connected to the ocean by narrow passages of water called straits. Gulfs can also have wide openings and are sometimes indistinguishable from larger bodies of water.
Major Gulfs
The Gulf of Mexico, bordered by the United States, Mexico, and the island nation of Cuba, is the world's largest gulf. It has a coastline of about 5,000 kilometers (3,100 miles). The Gulf of Mexico is connected to the Atlantic Ocean by the Straits of Florida, between Cuba and the U.S. state of Florida. It is connected to the Caribbean Sea by the Yucatán Channel, between Cuba and the Mexican peninsula of Yucatán.
The Gulf of Mexico is an important economic site for all three countries. The process of upwelling occurs near the Florida coast of the gulf, creating a rich variety of sea life. Upwelling is the process in which cold, nutrient-rich water from the bottom of the gulf is brought to the surface.
Fish and other organisms thrive in areas of upwelling. Commercial, sport, and recreational fishing thrive in the Gulf of Mexico. Oil deposits sit beneath the western Gulf of Mexico. Both Mexico (in the Bay of Campeche) and the U.S. (mainly around the coasts of Texas and Louisiana) have oil wells in the Gulf of Mexico.
The Gulf Stream, one of the most powerful ocean currents in the world, originates in the Gulf of Mexico. Gulf ports, including Houston, Texas; New Orleans, Louisiana; Veracruz, Mexico; and Havana, Cuba, continue to be important cities where goods are imported and exported by sea.
The Gulf of Mexico is also the site of strong storms. Hurricanes and other storms need warm water to develop. The Gulf of Mexico is a very warm body of water, so storms can often increase their strength. Cuba and Florida are regularly hit by hurricanes on their Atlantic and Gulf coasts.
Pollution also threatens life in the Gulf of Mexico. Oil shipping and drilling can spill tons of petroleum into the ecosystem. Two huge rivers, the Mississippi in the U.S. and the Grijalva in Mexico, empty into the gulf. Chemicals used for agriculture and industry have seeped into the water, helping to create one of the largest dead zones in the world. (A dead zone is a region where there is little oxygen or life beneath the surface of the ocean.)
River management has redirected the flow of the Mississippi River. Canals, dams, and drainage systems for agriculture and industry have provided power and irrigated land. They have also reduced the wetlands at the rivers mouth and delta. The Gulfs wetlands slow storms as they move toward land. The loss of these wetlands may have contributed to the destruction brought by Hurricane Katrina to the Gulf Coast between central Florida and Texas in 2005.
The Gulf of Carpentaria, on Australia's northeast coast, is an inlet of the Arafura Sea. Because the sea and the gulf are shallow, the exchange of water between the two is reduced. Sediment collects at the mouth of the gulf, forming underwater barriers. The low shore is bordered in some areas by wetlands and swamps.
This shallow gulf with a wide mouth creates the conditions for a yearly spectacle called the Morning Glory Cloud. In September and October, sea breezes from the Gulf of Carpentaria and the Arafura Sea meet and create an enormous, fast-moving cloud over the gulf. The Morning Glory Cloud can be 1,000 kilometers (621 miles) long and move at a rate of 60 kilometers per hour (37 miles per hour).
The Persian Gulf is an arm of the Arabian Sea bordered by Iran, Iraq, Kuwait, Saudi Arabia, Qatar, Bahrain, the United Arab Emirates, and Oman. Vast deposits of petroleum in this region make the Persian Gulf strategically important. Middle Eastern countries depend on the gulf for trade and for access to the Indian Ocean. All countries that consume oil from the region, including the U.S., have a vital interest in keeping the gulf open to shipping.
Additional Information
A gulf is any large coastal indentation. More specifically, such a feature is the reentrant of an ocean, regardless of size, depth, configuration, and geologic structure. The nomenclature for gulfs is far from uniform; names that may refer to sizable gulfs in various places include bay, bight, firth, sound, and fjord. In addition, a number of pronounced concavities of oceanic margins have no proper name at all. As such, many of the characteristics of gulfs may also apply to bays and other similar geographies.
This problem of nomenclature extends to the difference between gulfs and seas. There are many small seas, such as the Sea of Marmara (11,000 square km [about 4,200 square miles]) and the Sea of Azov (38,000 square km [about 14,700 square miles]), which, strictly speaking, are really gulfs of the ocean or other seas (the Sea of Azov is a gulf of the Black Sea). The Gulf of Aden (about 270,000 square km [about 104,000 square miles]), another example, is part of the Arabian Sea, and these water bodies have a common regime (similar tides, precipitation, evaporation, and so forth). The narrow sound of Bab el-Mandeb connects the gulf with the vast Red Sea (438,100 square km [about 169,000 square miles]) and exhibits a number of specific geomorphic features. The Red Sea in turn has two small gulfs to the north—namely, those of Suez and Aqaba.
The Bay of Bengal and the Arabian Sea are both gulfs, approximately the same size and having the same monsoonal water circulation. The Bay of Bengal is, however, the largest of the gulfs, with a surface area of 2,172,000 square km (838,600 square miles), a length of 1,850 km (1,150 miles), and a width of about 1,600 km (1,000 miles).
In some cases, the width of a gulf may exceed its length. The Great Australian Bight has the widest mouth (2,800 km [1,740 miles]). The Gulf of Guinea is the deepest; its maximum depth (6,363 metres [20,876 feet]) exceeds that of the Bay of Bengal by more than 1,000 metres (about 3,300 feet).
Topographic characteristics
Single gulfs usually are formed along linear shores of the continents. If the shoreline is irregular and has a complex geologic structure, groups of gulfs of a similar nature may occur. Most shorelines have small reentrants of various size that are called bays.
The shape and bottom topography of gulfs are amazingly diverse. They are determined by the geologic structure and development of the region. Homogeneous bedrock of low strength or resistance results in simple shapes and shallow depths. The Gulf of Riga (of the Baltic Sea) is a possible example of the type. Long, narrow arms with approximately parallel shores of the south Kara Sea extend inland for about 800 km (about 500 miles). They occupy troughs that originated by erosion during a period of lower sea level (Baidaratskaya Bay, Obskaya Bay with Tazovskaya Bay tributary, Yenisey Bay, Gydanskaya Bay). Deep, angular gulfs, on the other hand, are created along fractures, faults, and rifts (e.g., Varanger Fjord); they usually have irregular bottom topography. Parallel fractures form extremely deep, narrow gulfs with parallel shores, such as the Gulf of California. Genuine fjord-gulfs are notable for their very high length-to-width ratios (up to 50:1). In regions that have undergone nonuniform deformation and uplift, gulfs of complicated and irregular shape and bottom topography are consequently formed; the Gulf of St. Lawrence is an example.
Gulfs are connected with the sea by means of one or more straits. Sometimes there may be an archipelago in the mouth of the gulf, as in the Gulf of Bothnia. There are some gulfs that open into the sea or into another gulf on opposite sides (Baffin Bay, the Gulf of Aden, and the Gulf of Oman).
Factors that affect the characteristics of gulfs
Gulfs may differ from the adjacent ocean (or sea) by virtue of water properties and dynamics and processes of sedimentation. Such differences are determined by the size and the shape of a given gulf, by the depth and bottom topography, and, to a considerable extent, by the degree of isolation from the ocean. Climatic conditions also are important. Isolation from an adjacent ocean depends on the ratio of width of mouth to total surface area of a gulf or on the cross section of the mouth to total water volume. If there is a sill (a submarine ridge or rise), the ratio of depth above the sill to the depth of the gulf is of great importance. No extensive comparisons of these ratios have been made to date; hence, any analysis of controlling variables must remain somewhat qualitative.
A high sill hampers the water exchange between an ocean and gulf and may lead to stagnation (oxygen deficiency), as is found in some fjords of Norway, in the Red Sea, and, particularly, in the Black Sea. Also, the presence of a sill causes independent circulation of gulf waters, generated by local winds and the runoff of rivers. Sills are not indispensable for the formation of an independent circulation, however. A narrow mouth, as in the Gulf of Bothnia, leads to the same result.
In humid climates, the waters of gulfs are freshened by river runoff. Salinity is particularly low in the gulfs of the Baltic Sea and along the southern coast of the Kara Sea. Water becomes almost fresh in their heads, especially in the spring when snow begins to thaw. Gulfs of the arid zone suffer from intensive evaporation and receive little river runoff. Thus, salinity increases markedly in this climatic regime—up to 60 parts per thousand in the Persian Gulf and up to 350 parts per thousand in the Kara-Bogaz-Gol (a gulf of the Caspian Sea). In addition to its effect on salinity, river runoff delivers organic matter and nutrient salts that may determine the specific features of life in the gulfs. The number of genera and species of organisms is small, but the organisms present tend to develop in quantities. That is why shrimp, oyster, and other fisheries are concentrated in many gulfs.
Funnel-shaped gulfs, in which the depth gradually decreases headward, usually have resonant tides. The tidal range at the head of such gulfs is several times greater than that in the open ocean (e.g., Bristol Channel, Río de la Plata, Mezenskaya Bay, Shelikhova Gulf). The world maximum tidal range has been registered in the Bay of Fundy (18 metres [59 feet]). The regularity (magnitude and frequency) of the flood tide may be distorted in such instances, and the duration of the flood tide may become much shorter than that of the ebb tide. This may cause the phenomenon of tidal bore, in which a steep wave will move rapidly upstream for dozens of kilometres.
Gulfs of simple shape with a narrow mouth and a high degree of isolation from the ocean are often subject to seiches. These free oscillations can result from rapid changes of atmospheric pressure and, of course, from tectonic movements such as earthquakes. Seiches gradually decrease, but some oscillation continues long after their cause disappears. A high rise of the water (storm surge) occurs in long and shallow gulfs if winds from the sea are protracted. Such phenomena are difficult to predict, and the high water levels may cause floods. Seiches commonly occur at the heads of Helgoländer Bay in the North Sea and in the Gulf of Finland.
Certain aspects of sedimentation are affected by the isolation of gulfs from the ocean and river runoff. The rate of sediment accumulation in gulfs of limited area may be very high. This, of course, is a function of river discharge; sediment composition is usually similar to that of the load transported by entering rivers. Deposition of calcium carbonate often occurs in shallow gulfs in the arid zones where few if any perennial streams exist. The bottoms of long gulfs (or gulfs having sills) are usually covered with silt even at the shallowest depths (e.g., Hudson Bay, the Bo Hai [Gulf of Chihli], the inlets or gubas of the Kara Sea, the Gulf of Riga). Only strong tidal currents can prevent this siltation and, in some cases, cause the opposite phenomenon of bottom erosion. Currents maintain the existence of or actively deepen bottom troughs in narrow-mouthed gulfs whose depths are more than 200 metres (about 660 feet), whereas depths of adjacent parts of the open ocean are only on the order of some dozens of metres.
Waves of the open ocean either do not penetrate into comparatively isolated gulfs or—if they do—they become greatly reduced after entry. Small local waves that are related to gulf size prevail there. This tends to make gulfs quite navigable, and seaports and harbours have generally been situated on them.
Classification of gulfs
The geologic structure and developmental history of gulfs are as varied as are those of the continents or oceans proper. The factors discussed above influence the morphological peculiarities of gulfs, and the latter in turn permit some general division or classification of these features to be made. The several groups in one possible scheme are discussed here using typical gulfs of each group as examples.
Areas situated in open concavities of the continental coast (Gulf of Alaska, Bay of Biscay, Gulf of Guinea, Great Australian Bight, Bay of Bengal, Gulf of Tehuantepec, for example) are classified as the A1 group. The depth of these gulfs in the region of the mouth usually is on the order of kilometres. The continental shelf and continental slope are generally pronounced. The general shape of such gulfs is simple; width of mouth usually exceeds its length. Water circulation and its physical properties are similar to those of the oceans. The character of the marine faunas does not differ from that of oceanic areas.
Large areas considerably isolated from oceans, such as the Gulf of Mexico and Baffin Bay, are designated as group A2. The former includes a geosynclinal hollow, founded in the Mesozoic Era (251 million to 65.5 million years ago) and finally shaped during the Paleogene and Neogene periods (65.5 million to 2.6 million years ago). It is connected with the ocean by the narrow and relatively shallow Straits of Florida and the Yucatán Channel. Baffin Bay is a rift hollow that is connected by straits with the Atlantic.
Ocean gulfs, such as the Gulfs of Oman, California, Aden, and some others, have smaller areas and are isolated to a lesser degree. These features, in group A3, have shapes that are determined by young faults and fractures. Depths in these gulfs generally exceed 1 kilometre (0.6 mile). Unlike the previous group, in which gulfs might be of composite geologic structure, these occupy areas that have undergone only a single episode of deformation.
Gulfs situated on the continental shelf, such as the Bay of Fundy, Hudson Bay, Río de la Plata, San Matías Gulf (off Argentina), and others, are in group B. The depth of such gulfs is up to 200 metres (about 660 feet) or more, and their configuration is determined by geologic conditions. Because shelf areas repeatedly became dry land when the sea level fell during the ice ages, these gulfs received their final shape during the Pleistocene Epoch. The Gulf of St. Lawrence is included in this group, though it is really intermediate between groups A3 and B. It contains both a pronounced shelf and a long trough up to 530 metres (1,740 feet) deep.
Gulfs of intercontinental and marginal seas are considered to be a third category. These may be divided into group C1, which consists of gulfs of basin seas, including the deepwater part only (Gulf of Aqaba) or both the deepwater and the shelf parts (Gulf of Honduras), and group C2, the shelf gulfs of the same seas (e.g., the Persian Gulf, the Gulf of Suez, Anadyrsky Gulf, the Bristol and Norton channels, and Shelikhova Gulf).
Finally, there are the gulfs of the shelf seas (gubas of the Arctic seas of Russia, gulfs of the Baltic and the White Seas, the Gulf of Carpentaria, the Bo Hai, and many others), which are placed in group D. The shallow character of the shelf seas influences the water dynamics of the gulfs. Water exchange is weakened, and sediments may accumulate in the gulf mouths, thus forming submarine barriers and further reducing exchange.

#19 Dark Discussions at Cafe Infinity » Close Quotes - VI » 2025-09-15 17:21:09
- Jai Ganesh
- Replies: 0
Close Quotes - VI
1. There's a gap between what I want to do, what I do on camera, and what gets edited. Right? So the goal is to try and close the gaps. What's the biggest compliment is if I read a review and it's exactly what I wrote down in my diary before ever filming it. That's really cool. That's the biggest signifier of closing the gaps. - Matthew McConaughey
2. I have very few friends. I have a handful of close friends, and I have my family, and I haven't known life to be any happier. - Brad Pitt
3. Eradications are special. Zero is a magic number. You either do what it takes to get to zero and you're glad you did it; or you get close, give up and it goes back to where it was before, in which case you wasted all that credibility, activity, money that could have been applied to other things. - Bill Gates
4. Happiness, for me, has to be real - life that is made of real conversations, of spending quality time with close friends, walks in nature and woods, praying, feeling real gratitude, reading good books, being able to be in the moment and hearing the sounds of nature. - Bhumika Chawla
5. We are not even close to finishing the basic dream of what the PC can be. - Bill Gates
6. I barely need to reiterate what you already know: the close links that exist between our people and the people of Venezuela and Hugo Chavez, the promoter of the Bolivarian Revolution and the United Socialist Party he founded. - Fidel Castro
7. One sees qualities at a distance and defects at close range. - Victor Hugo
8. I don't think there is a perfect athlete. But if I had to come close to picking someone who demonstrates all the traits that I feel an athlete should have, I would say the perfect athlete would be Tiger Woods. He has the ability, he's humble and he's very good at what he does. - Jackie Joyner-Kersee.
#20 Jokes » Love Jokes - II » 2025-09-15 16:57:58
- Jai Ganesh
- Replies: 0
Q: What is love?
A: The delusion that one woman differs from another.
* * *
Q: How did the girl get a prince to fall in love with her?
A: She wore a raspberry beret.
* * *
Q: Why shouldn't you marry a tennis player?
A: Because love means nothing to them!
* * *
Q: How do you turn a fox into an elephant?
A: Marry it.
* * *
Q: What did the toaster say to the slice of bread?
A: I want you inside me!
* * *
#21 Science HQ » Bismuth » 2025-09-15 16:22:46
- Jai Ganesh
- Replies: 0
Bismuth
Gist
Bismuth (symbol Bi, atomic number 83) is a brittle, silvery-white post-transition metal with low toxicity that is used in alloys, electronics, pharmaceuticals (like Pepto-Bismol), and paints. Recovered as a by-product of other metals, bismuth's unique bismuth crystal structure and chemical properties lead to its common use in various industrial and medical applications.
Bismuth finds its main uses in pharmaceuticals, atomic fire alarms and sprinkler systems, solders and other alloys and pigments for cosmetics, glass and ceramics.
Summary
Bismuth is a chemical element; it has symbol Bi and atomic number 83. It is a post-transition metal and one of the pnictogens, with chemical properties resembling its lighter group 15 siblings antimony. Elemental bismuth occurs naturally, and its sulfide and oxide forms are important commercial ores. The free element is 86% as dense as lead. It is a brittle metal with a silvery-white color when freshly produced. Surface oxidation generally gives samples of the metal a somewhat rosy cast. Further oxidation under heat can give bismuth a vividly iridescent appearance due to thin-film interference. Bismuth is both the most diamagnetic element and one of the least thermally conductive metals known.
Bismuth was formerly understood to be the element with the highest atomic mass whose nuclei do not spontaneously decay. However, in 2003 it was found to be very slightly radioactive. The metal's only primordial isotope, bismuth-209, undergoes alpha decay with a half-life roughly a billion times longer than the estimated age of the universe.
Bismuth metal has been known since ancient times. Before modern analytical methods bismuth's metallurgical similarities to lead and tin often led it to be confused with those metals. The etymology of "bismuth" is uncertain. The name may come from mid-sixteenth-century Neo-Latin translations of the German words weiße Masse or Wismuth, meaning 'white mass', which were rendered as bisemutum or bisemutium.
Bismuth compounds account for about half the global production of bismuth. They are used in cosmetics; pigments; and a few pharmaceuticals, notably bismuth subsalicylate, used to treat diarrhea. Bismuth's unusual propensity to expand as it solidifies is responsible for some of its uses, as in the casting of printing type.[ Bismuth, when in its elemental form, has unusually low toxicity for a heavy metal. As the toxicity of lead and the cost of its environmental remediation became more apparent during the 20th century, suitable bismuth alloys have gained popularity as replacements for lead. Presently, around a third of global bismuth production is dedicated to needs formerly met by lead.
Details
Bismuth (Bi) is the most metallic and the least abundant of the elements in the nitrogen group (Group 15 [Va] of the periodic table). Bismuth is hard, brittle, lustrous, and coarsely crystalline. It can be distinguished from all other metals by its colour—gray-white with a reddish tinge.
Element Properties:
atomic number : 83
atomic weight : 208.98040
melting point : 271.3 °C (520.3 °F)
boiling point : 1,560 °C (2,840 °F)
density : 9.747 gram/{cm}^{3} at 20 °C (68 °F)
oxidation states : +3, +5
History
Bismuth evidently was known in very early times, since it occurs in the native state as well as in compounds. For a long period, however, it was not clearly recognized as a separate metal, having been confused with such metals as lead, antimony, and tin. Miners during the Middle Ages apparently believed bismuth to be a stage in the development of silver from baser metals and were dismayed when they uncovered a vein of the metal thinking they had interrupted the process. In the 15th-century writings of the German monk Basil Valentine this element is referred to as Wismut, a term that may have been derived from a German phrase meaning “white mass.” In any case it was Latinized to bisemutum by the mineralogist Georgius Agricola, who recognized its distinctive qualities and described how to obtain it from its ores. Bismuth was accepted as a specific metal by the middle of the 18th century, and works on its chemistry were published in 1739 by the German chemist Johann Heinrich Pott and in 1753 by the Frenchman Claude-François Geoffroy.
Occurrence and distribution
Bismuth is about as abundant as silver, contributing about 2 × {10}^{-5} weight percent of Earth’s crust. Its cosmic abundance is estimated as about one atom to every 7,000,000 atoms of silicon. It occurs both native and in compounds. In the native state, it is found in veins associated with lead, zinc, tin, and silver ores in Bolivia, Canada, England, and Germany. Its naturally occurring compounds are chiefly the oxide (bismite or bismuth ochre, Bi2O3), the sulfide (bismuthinite or bismuth glance, Bi2S3), and two carbonates (bismutite, (BiO)2CO3, and bismutosphaerite). Commercial bismuth, however, is produced largely as a by-product in the smelting and refining of lead, tin, copper, silver, and gold ores. Thus, it comes—for example—from tungsten ores in South Korea, lead ores in Mexico, copper ores in Bolivia, and both lead and copper ores in Japan. By the early 21st century, however, China was leading the world in both the mining and the refining of bismuth. Pure bismuth can also be obtained by reducing the oxide with carbon or by roasting the sulfide in the presence of charcoal and metallic iron to remove the sulfur.
Bismuth forms only one stable isotope, that of mass 209. A large number of radioactive isotopes are known, most of them very unstable.
Commercial production and uses
Bismuth is volatile at high temperature, but it usually remains with the other metals after smelting operations. Electrolytic refining of copper leaves bismuth behind as one component of the anode sludge. Separation of bismuth from lead by the Betterton–Kroll process involves the formation of high-melting calcium or magnesium bismuthide (Ca3Bi2 or Mg3Bi2), which separates and can be skimmed off as dross. The dross may be chlorinated to remove the magnesium or calcium, and finally the entrained lead. Treatment with sodium hydroxide then produces highly pure bismuth. An alternative separation, the Betts process, involves electrolytic refining of lead bullion (containing bismuth and other impurities) in a solution of lead fluosilicate and free fluosilicic acid, bismuth being recovered from the anode sludge. Separation of bismuth from its oxide or carbonate ores can be effected by leaching with concentrated hydrochloric acid. Dilution then precipitates the oxychloride, BiOCl. This, on heating with lime and charcoal, produces metallic bismuth.
Metallic bismuth is used principally in alloys, to many of which it imparts its own special properties of low melting point and expansion on solidification (like water and antimony). Bismuth is thus a useful component of type-metal alloys, which make neat, clean castings; and it is an important ingredient of low-melting alloys, called fusible alloys, which have a large variety of applications, especially in fire-detection equipment. A bismuth–manganese alloy has been found effective as a permanent magnet. Small concentrations of bismuth improve the machinability of aluminum, steel, stainless steels, and other alloys and suppress the separation of graphite from malleable cast iron. Thermoelectric devices for refrigeration make use of bismuth telluride, Bi2Te3, and bismuth selenide, Bi2Se3. Liquid bismuth has been used as a fuel carrier and coolant in the generation of nuclear energy.
The principal chemical application of bismuth is in the form of bismuth phosphomolybdate (BiPMo12O40), which is an effective catalyst for the air oxidation of propylene and ammonia to acrylonitrile. The latter is used to make acrylic fibres, paints, and plastics. Pharmaceutical uses of bismuth have been practiced for centuries. It is effective in indigestion remedies and antisyphilitic drugs. Slightly soluble or insoluble salts are utilized in the treatment of wounds and gastric disorders and in outlining the alimentary tract during X-ray examination, and bismuth is sometimes injected in the form of finely divided metal, or as suspensions of its insoluble salts. Substantial quantities of the oxychloride, BiOCl, have been used to impart a pearlescent quality to lipstick, nail polish, and eye shadow.
Properties and reactions
Bismuth is a rather brittle metal with a somewhat pinkish, silvery metallic lustre. Bismuth is the most diamagnetic of all metals (i.e., it exhibits the greatest opposition to being magnetized). It is hard and coarsely crystalline. It undergoes a 3.3 percent expansion when it solidifies from the molten state. Its electrical conductivity is very poor, but somewhat better in the liquid state than in the solid. With respect to thermal conductivity, it is the poorest of all metals except mercury.
Although it does not tarnish in air at ordinary temperatures, bismuth forms an oxide coating when heated and is oxidized rapidly at its boiling point of 1,560 °C. The yellow colour of this oxide distinguishes it from those formed by other metals. At red heat, bismuth reacts with steam, but it is not affected by cold, air-free water; it combines directly with sulfur and with the halogens (fluorine, chlorine, bromine, iodine). The element is not attacked by hydrochloric acid, and only slightly by hot sulfuric acid, but it is rapidly dissolved by either dilute or concentrated nitric acid.
Bismuth atoms have the same electronic structure in their outermost shell as do the other elements of the nitrogen group. They can, therefore, form three single covalent bonds, exhibiting either a +3 or −3 oxidation state. The element has a somewhat lower electronegativity than the others, and its lone pair of electrons is evidently quite inert, causing the +5 state of bismuth to be rare and unstable.
Analytical and physiological chemistry
Bismuth is usually determined gravimetrically, being precipitated and weighed as the phosphate or the oxychloride, BiOCl. To produce the latter, a suitable amount of hydrochloric acid is added to a nitric acid solution containing the bismuth, and the resulting solution is poured into a large volume of water, causing the oxychloride to precipitate. Volumetric and colorimetric methods of determination are also available.
Bismuth is relatively nontoxic, the least so of the heavy metals. It is generally not an industrial hazard. Although bismuth and certain of its compounds find considerable therapeutic use, some authorities recommend that other remedies be substituted. Soluble inorganic bismuth compounds are toxic.
Additional Information:
Appearance
Bismuth is a high-density, silvery, pink-tinged metal.
Uses
Bismuth metal is brittle and so it is usually mixed with other metals to make it useful. Its alloys with tin or cadmium have low melting points and are used in fire detectors and extinguishers, electric fuses and solders.
Bismuth oxide is used as a yellow pigment for cosmetics and paints, while bismuth(III) chloride oxide (BiClO) gives a pearly effect to cosmetics. Basic bismuth carbonate is taken in tablet or liquid form for indigestion as ‘bismuth mixture’.
Biological role
Bismuth has no known biological role, and is non-toxic.
Natural abundance
Bismuth occurs as the native metal, and in ores such as bismuthinite and bismite. The major commercial source of bismuth is as a by-product of refining lead, copper, tin, silver and gold ores.
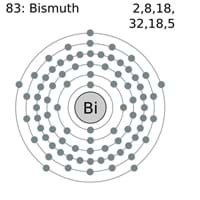
#22 Re: Jai Ganesh's Puzzles » General Quiz » 2025-09-15 15:40:25
Hi,
#10555. What does the term in Geography Borough mean?
#10556. What does the term in Geography Boundary mean?
#23 Re: Jai Ganesh's Puzzles » English language puzzles » 2025-09-15 15:23:34
Hi,
#5749. What does the noun inlet mean?
#5750. What does the adjective innate mean?
#24 Re: Jai Ganesh's Puzzles » Doc, Doc! » 2025-09-15 15:11:18
Hi,
#2468. What does the medical term Axonotmesis mean?
#25 Re: Jai Ganesh's Puzzles » 10 second questions » 2025-09-15 14:40:36
Hi,
#9734.